
Back Grondtoon Afrikaans تردد أساسي Arabic Əsas tonun tezliyi Azerbaijani মৌলিক কম্পাঙ্ক Bengali/Bangla Freqüència fonamental Catalan Základní frekvence Czech Grundfrekvens Danish Grundfrequenz German Θεμελιώδης συχνότητα Greek Fundamenta frekvenco Esperanto
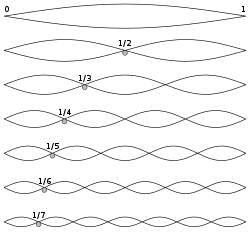
The fundamental frequency, often referred to simply as the fundamental (abbreviated as f0 or f1 ), is defined as the lowest frequency of a periodic waveform.[1] In music, the fundamental is the musical pitch of a note that is perceived as the lowest partial present. In terms of a superposition of sinusoids, the fundamental frequency is the lowest frequency sinusoidal in the sum of harmonically related frequencies, or the frequency of the difference between adjacent frequencies. In some contexts, the fundamental is usually abbreviated as f0, indicating the lowest frequency counting from zero.[2][3][4] In other contexts, it is more common to abbreviate it as f1, the first harmonic.[5][6][7][8][9] (The second harmonic is then f2 = 2⋅f1, etc. In this context, the zeroth harmonic would be 0 Hz.)
According to Benward's and Saker's Music: In Theory and Practice:[10]
Since the fundamental is the lowest frequency and is also perceived as the loudest, the ear identifies it as the specific pitch of the musical tone [harmonic spectrum].... The individual partials are not heard separately but are blended together by the ear into a single tone.
- ^ Nishida, Silvia Mitiko. "Som, intensidade, frequência". Instituto de Biociências da Unesp. Retrieved 2024-09-05.
- ^ "sidfn". Phon.UCL.ac.uk. Archived from the original on 2013-01-06. Retrieved 2012-11-27.
- ^ Lemmetty, Sami (1999). "Phonetics and Theory of Speech Production". Acoustics.hut.fi. Retrieved 2012-11-27.
- ^ "Fundamental Frequency of Continuous Signals" (PDF). Fourier.eng.hmc.edu. 2011. Archived from the original (PDF) on 2014-05-14. Retrieved 2012-11-27.
- ^ "Standing Wave in a Tube II – Finding the Fundamental Frequency" (PDF). Nchsdduncanapphysics.wikispaces.com. Archived from the original (PDF) on 2014-03-13. Retrieved 2012-11-27.
- ^ "Physics: Standing Waves". Physics.Kennesaw.edu. Archived from the original (PDF) on 2019-12-15. Retrieved 2012-11-27.
- ^ Pollock, Steven (2005). "Phys 1240: Sound and Music" (PDF). Colorado.edu. Archived from the original (PDF) on 2014-05-15. Retrieved 2012-11-27.
- ^ "Standing Waves on a String". Hyperphysics.phy-astr.gsu.edu. Retrieved 2012-11-27.
- ^ "Creating musical sounds". OpenLearn. Open University. Archived from the original on 2020-04-09. Retrieved 2014-06-04.
- ^ Benward, Bruce and Saker, Marilyn (1997/2003). Music: In Theory and Practice, Vol. I, 7th ed.; p. xiii. McGraw-Hill. ISBN 978-0-07-294262-0.