
Back مجموعة محدبة Arabic Convexidá AST Conjunt convex Catalan Konvexní množina Czech Мăкăрăлчăк йыш CV Konvexe Menge German Κυρτό σύνολο Greek Convex set English Konveksa aro Esperanto Convexidad Spanish
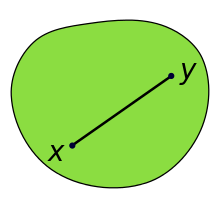

In Euclidean space, a region is a convex set if the following is true. For any two points inside the region, a straight line segment can be drawn. If every point on that segment is inside the region, then the region is convex.
The point is that a convex curve forms the boundary of a convex set. So, any shape which is concave, or has a hollow, cannot be a convex set.